Are Humans Rational?
(Singularly So!)

General Orientation
Texts/Readings
Syllabus
Slide Decks etc., Meeting by Meeting

General Orientation

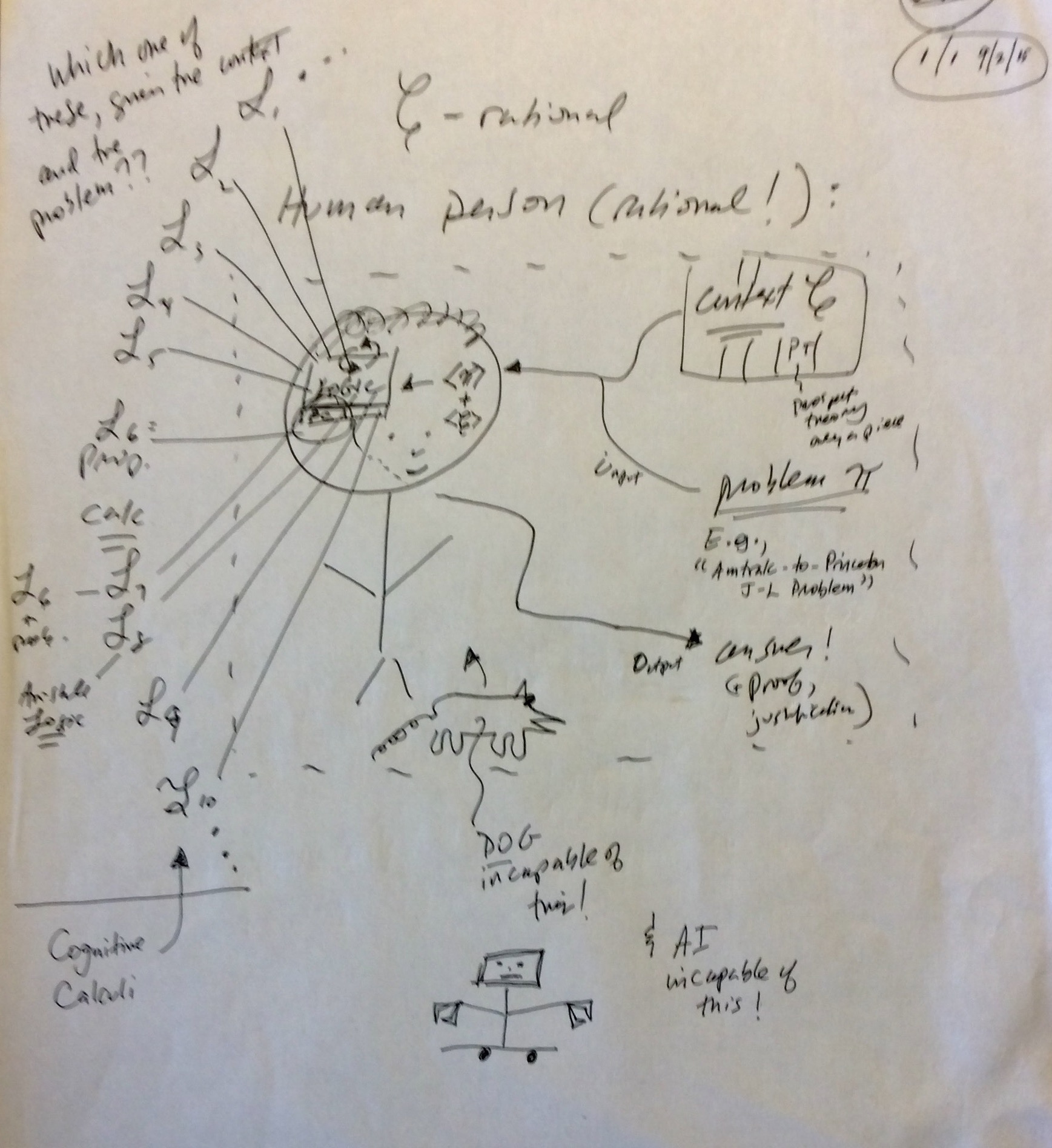
The Aristotelian dictum that we are rational animals is under severe attack these days. In fact, the previous sentence may be to seriously understate the situation: the dictum is perhaps outright rejected by many, if not most. From psychologists of reasoning and decision-making to behavioral economists to the “new atheists” (all groups whose message we will consider in this class), the onslaught is firmly underway, and fierce. Yet this course revolves around a defense of the proposition that Aristotle, modernized along Leibnizian, Piagetian, and Bringsjordian $\times$ 2 lines, is right. This proposition, put a bit more precisely, is:

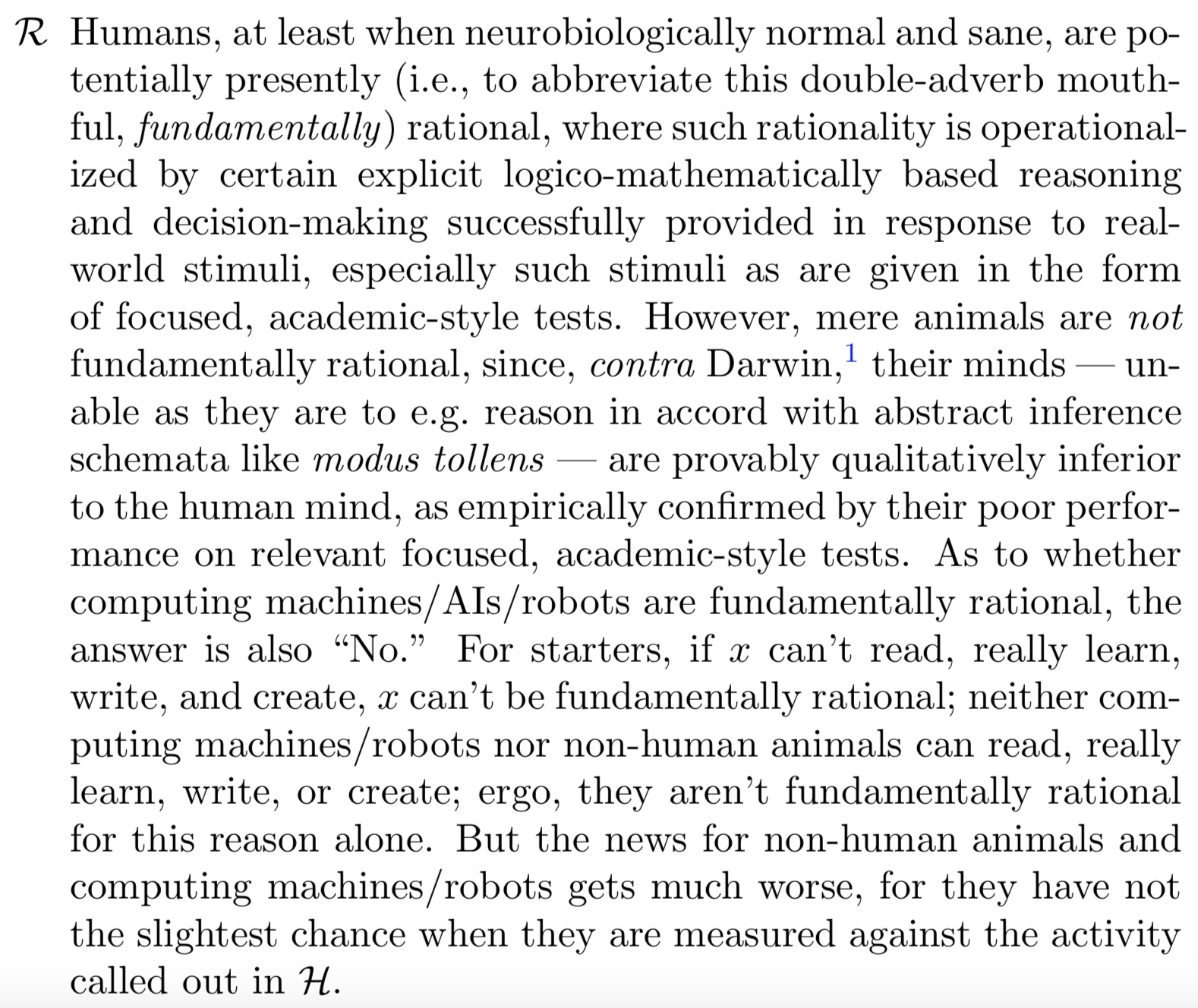
$\mathcal{R}$

Humans, at least neurobiologically normal adult ones, are fundamentally rational, where rationality is constituted by certain logico-mathematically based reasoning and decision-making in response to real-world stimuli, including stimuli given in the form of focused tests; but mere animals are not fundamentally rational, since, contra Darwin, their minds are fundamentally qualitatively inferior to the human mind. As to whether computing machines/robots are fundamentally rational, the answer is “No.” For starters, if $x$ can’t read, write, and create, $x$ can’t be rational; computing machines/robots can neither read nor write nor create; ergo, they aren’t fundamentally rational. But the news for non-human animals and computing machines/robots gets much worse, for they have not the slightest chance when they are measured against $\mathcal{H}$, which runs as follows:
$\mathcal{H}$

Humans have the ability to gain knowledge by reasoning (e.g., deductively) quantificationally and recursively over abstract concepts, including abstract concepts of a highly expressive, including infinitary, nature, expressed in arbitrarily complex natural and formal languages.
Rapid Example

For a rapid (finitary) example (provided long ago on Amtrak to Selmer by Professor Yingrui Yang, who relayed it from Professor Johnson-Laird) of some of the stimuli to which $\mathcal{R}$ refers:
- Amtrak-to-Princeton J-L Problem
  Suppose that the following two statements are true:
  1. Everyone likes anyone who likes someone.
  2. Abigail likes Bruno.
  Does it follow deductively that everyone likes Bruno? Prove that your answer is right!
Notice: ‘Fundamentally’

Notice that the adverb ‘fundamentally’ is used repeatedly in $\mathcal{R}$. This means, among other things, that humans are \emph{potentially} rational. What humans need in order to reason and make decisions in the relevant ways, we (i.e., SB & AB) further claim, is sustained study of the relevant logic and mathematics, and an ability to use what one has studied in order to reason and decide correctly in response to the aforementioned stimuli. In the course of our defense, we’re going to supply at least some of the relevant logic and mathematics to you. Hence, as you receive and judge our case, we believe that you will move some distance from being merely fundamentally rational to being presently rational. We believe it’s fair to say that the purpose of college is to markedly increase the level of reasoning and decision-making power that constitutes being presently rational.
Context: A Research University

You have wisely decided to attend a technical research university, with a faculty-led mission to create new knowledge and technology in collaboration with students. RPI is the oldest such place in the English-speaking world; it may know a thing or two about this mission. The mission drives those who teach you in this class. The last thing we want to do is simply convey to you how others answer the driving question that gives this class its name. As should be obvious by now, we think we have correct answers to the driving question, and are working hard to explain them, specify them formally, and disseminate them. We’ll tell you objectively what other thinkers say, but we’re going to tell you that, at least for the most part, they’re wrong, and why they’re wrong by supplying suitable arguments. (As an immediate example, we hereby inform you that Ernest Sosa has long claimed that rationality should not be measured against formal logic; see his “Are Humans Rational?"¹, which we shall discuss in class.) You can judge whether our arguments are sound or not. And you should start to develop your own individual answer, which may well be different than ours. You should seek to defend your answer, and will indeed by asked to do so in this class. For purposes of evaluating your performance, it matters not a whit what your positions is; what matters is your understanding of the technical material presented, and the quality of your reasoning given in defense of your positions.
A Disclaimer!

Please note that guest lecturers other than A Bringsjord should not be assumed to have affirmed anything like the claims $\mathcal{R}$ and $\mathcal{H}$ issued above. This thus applies specifically to, in the 2019 edition of AHR?, TA xxxx xxxx, Mike Giancola, and any other guest researchers from the RAIR Lab who visit us to speak/demo. It also applies to those who have helped in the past editions; e.g. Dan Arista, Professors John Milanese and John Licato, Thomas Carter, Atriya Sen, etc. As to what these thinkers hold in connection with $\mathcal{R}$, that is an open question. You are free to inquire.
Graduate Teaching Assistants; Further Help

The TA for the F2019 edition course is: Can Mekik; email address: can.mekik@gmail.com. Can will hold office hours in the Cognitive Architecture Lab (41 9th St, Floor 1; aka EMPAC Annex; requires card access; ring bell/knock on front window to get in), on Thurdays from 1pm to 3pm, and by appointment. Additional assistance will be provided by RAIR-Lab researcher and PhD student Mike Giancola, who specializes in some of the topics discussed in the course (e.g. AIs that can rationally handle inconsistencies arising in real-life scenarios).

Please note again A Disclaimer!.
Prerequisites

There are no formal prerequisites. However, this course covers parts of such things as formal deductive logic, formal probabilistic logic, game theory, etc. This implies that — for want of a better phrase — students are expected to have a degree of mathematical maturity. At RPI, this expectation is quite reasonable.

To be a bit more specific, the logico-mathematics alluded to in claims $\mathcal{R}$ and $\mathcal{H}$ can be partitioned into three general areas: analysis and continuous mathematics (A1); deductive formalisms, systems, and techniques (A2); and inductive/statistical/probabilistic formalisms, systems, and techniques (A3). Because of the nature of RPI’s requirements for a BS, A1 is generally already covered in other classes (on the integral and differential calculus). The emphasis in the present class is on (introductory elements of) areas A2 and A3.

Texts/Readings

In-class lectures deliver crucial content. (Assuming that things go according to plan, all lectures will be recorded, and will be available for review to all students.) Attendance is required and note-taking is key. Sometimes slides will be distributed by email. Most readings will be electronic, and either distributed by email, or can be obtained by url. As a first example, students should read Nicholson Baker’s “The Wrong Answer” asap, since it (we claim) represents a stark example of an implicit denial of both $\mathcal{R}$ and $\mathcal{H}$. As to books, it’s required that students purchase and read Kahneman’s (2013) Thinking, Fast and Slow. It’s recommended that students read the available-online The Nature of Rationality by Robert Nozick; S Bringsjord will be drawing from this book at times (in ways that will be announced clearly). Finally, five class meetings will each draw directly from a chapter in S Bringsjord’s forthcoming G\"{o}del’s Great Theorems, from Oxford University Press.

Syllabus

The Fall 2019 PDF version available here. Recall that the syllabus has hotlinks to some required reading!

Slide Decks etc., Meeting by Meeting

Setting the Stage
- August 29 2019: The syllabus was projected and presented, course overview, etc. (Selmer Bringsjord)
- September 3 2019: Setting the Stage; $\mathcal{R}$ and $\mathcal{H}$ Discussed (Selmer Bringsjord)
The Attack on Rationality from Failures of Deductive Reasoning
- September 5 2019: Some “Classic” Shots at Main Claim $\mathcal{R}$ (Selmer Bringsjord)
- September 9 2019: Rational Analysis of Shots at Main Claim $\mathcal{R}$ (Selmer Bringsjord)
- September 12 2019: “Cognitive” Deductive Shots @ $\mathcal{R}$ (Selmer Bringsjord)
The Attack on Rationality from Failures of Inductive/Probabilistic Reasoning
- September 16 2019:
  - Re The Monty Hall Problem (Selmer Bringsjord)
  - “Artilects” and the Mechanisms of Cognition (Mike Giancola)
- September 19 2019: Addenda to Prop Calc; Selmer’s Monty Fall Problem; The Case of Linda, & Some Probability Formalisms (Selmer Bringsjord)
Refuting Kahneman on Rationality — Again
- September 23 2019: Efficient! Critique of Kahneman on Investing (Selmer Bringsjord)
Test 1
- September 26 2019: Test 1 (Selmer Bringsjord)
- The solutions have been found and elegantly expressed by Can Mekik.
The Meaning of Life, Rationally Considered
- September 30 2019: Rational Investigation of … The Meaning (if any) of Life (Mike Giancola & Selmer Bringsjord)
A Rational Treatment of AI & Machine Learning
- October 3 2019 The Singularity, Rationally Considered (Mike Giancola & Selmer Bringsjord)
- October 7 2019 The Future of AI: G$\"{o}$del’s Either/Or; AI, Consciousness, and Westworld; Machine Learning (Selmer Bringsjord)
  
  This class includes a presentation and discussion revolving around the 2018 paper “Do Machine-Learning Machines Learn?” by Bringsjord, S., Govindarajulu, N.S., Banerjee, S. & Hummel, J., which is in Müller, V., ed., Philosophy and Theory of Artificial Intelligence 2017 (Berlin, Germany: Springer SAPERE), pp. 136–157, Vol. 44 in the book series. The paper answers the question that is its title with a resounding No.
- October 10 2019 The MiniMaxularity & Human Disemployment (Selmer Bringsjord)
- October 17 2019 Logicist Machine Ethics Can Save Us (Selmer Bringsjord & Mike Giancola)
Rationality Handles Paradoxes and Inconsistency
- October 21 2019 Paradox — Even in Jets
  This class revolves around handling inconsistency, and includes, first, some initial paradoxes that appear to force us into contradictions (see the first slide immediately below), and following on that, analysis of a real-life case of a paradox that takes a jet down, and ends the life of two unfortunate pilots.
  - Rationality & Paradox, Part I: The Liar; The Barber; Dr Who Saves the Day; The Knowability Paradox (S Bringsjord)
  - The Dangers of Inconsistency (Mike Giancola & Selmer Bringsjord)
Test 2
- October 24 2019 Test 2
Rationality Handles Paradoxes and Inconsistency — continued
- October 28 2019: Newcomb’s Problem: One-Box XOR Two-Box, Which is Rational? (Selmer Bringsjord)
- October 31 2019: Solving the Lottery Paradox — and the St Petersburg Paradox (Selmer Bringsjord & Mike Giancola)
- November 4 2019 An Asymmetry in the Multiverse “Escape” From The Paradoxes of Time Travel (Selmer Bringsjord)
Darwin’s Dumb Ideas
- November 7 2019 Contra Darwin, Humans are Rational Animals, But Mere Animals are Not; and Darwin is Irrational in Thinking Otherwise (Selmer Bringsjord)
- November 11 2019: Formalizing and Demonstrating Discontinuity (in the context of PHP’s BBS Paper “Darwin’s Mistake”) (Selmer Bringsjord)
Animals and AIs: Can They Talk? — Because if not they’re not capable of being rational …
- November 14 2019: NLP: Animals, Machines, and Money (Selmer Bringsjord)
- November 18 2019 Informal Intro to “Gold-style” Learning, Extended; A RAIR-Lab Target
Is Religious Belief Rational?
- November 21 2019: On “Breaking the Spell” of Irrationality; A Better Version of Pascal’s Wager (Selmer Bringsjord)
G\"{o}delian Steeples of Rationalism
- November 25 2019: Steeple #1: Godel’s Completeness Theorem (Selmer Bringsjord)
- December 2 2019: No class: snowpocalypse. (At times like this, with alpine paradise bordering the Capital District, one wonders: Why ski West??)
- December 5 2019: Steeple #2: Godel’s Incompleteness Theorem (with: Can a machine match this?) (Selmer Bringsjord)
- December 8 2019: Steeple #3: Godel’s “Silver Blaze” Theorem (Selmer Bringsjord)

Footnotes:

Sosa, E. (1999) “Are Humans Rational?” in Cognition, Agency and Rationality, K. Korta, E. Sosa, \& X. Arrazola, eds., (Dordrecht, The Netherlands: Kluwer), pp. 1–8. This book is the Proceedings of the Fifth International Colloquium on Cognitive Science.

Are Humans Rational? (Singularly So!)

Table of Contents